注意:理解曲线系最重要的要点是将某方程F(x,y)=0中的多项式F(x,y)当做关于一点坐标的函数.当一点(m,n)在F(x,y)=0的图像上时,便满足F(m,n)=0 1.先从一道不能算曲线系精髓的直线系题目说起 如图AD平分∠BAC,AD上任取一点E,使得BE交边CD于F,CE交边BD于G 求证AD平分∠GAE ……

前言 最近感觉看睿站有点多，浪费了很多时间。 正巧看见了rsshub的b站up订阅功能 就开始寻找rss服务 但找了半天都没有合适的服务 决定自己建一个 准备 服务器一台 一定的动手能力、搜索能力 过程 一开始使用Docker镜像的方式进行安装 使用SSH进行安装 sudo apt instal……

啊，中国的朋友们大家好，我是来自HHS的XGN。最近冠状病毒爆发，各地都在出台政策防治。笔者刚从老家回来，路上受到了各种检查和测温。假设我们将每一台车上的人看作一个服务器，每一组检查站看成一个客户端。那么，检查站想要连续、精确的获取到车上的人的体温，有什……

今天TM研究了一天这个破烂问题。 问题本质是git不走系统代理，再加上Github Desktop又不支持手动设置代理，只知道憨憨的调用git命令。解决问题的方法非常简单，就是设置git代理： 在命令行中输入(确保git在目录或path下)： git config --global https.proxy 127.0.0.1……

$1.\int{\frac{1}{\sqrt{x^2±a^2}}}dx=ln|x+\sqrt{x^2+a^2}|+C(a>0)$ $1.5.特别地, \int{\frac{1}{\sqrt{x(x+a)}}}dx=ln(\sqrt{x}+\sqrt{x+a})+C(a>0)$ $2.\int{tan^2(x)}dx=tanx-x+C$ $3.\int{\frac{1}{x^2+a^2}}dx=\frac{arctan{(\frac{x}{a})}}{a}+C$ $4.\int{\fra……

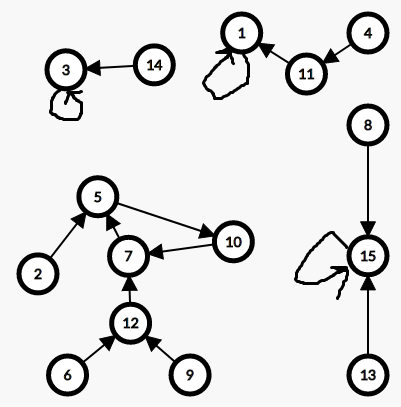
OGF - Cayley Formula Question Statement Prove # of trees on [n] = n^{n-2} Solution f:(1,2,3,...,n)\to(1,2,3,...,n) f(1)=1,f(n)=n Functional Digraph(directed graph) D V(D)=[n] 编者注：V为节点集合 (i,j)\in E(D) if f(i)=j 注：E为边集合 Example | ……

$\mathcal A$ is a collection of combinatorial objects. => $\mathcal A$ and $\mathcal B$ are combinatorically isomorphic if there exist a size-preserving bijection between $\mathcal A$ and $\mathcal B$ Q1 Prove that number of Dyck Paths D_{n-1} equa……

阅读本文前可以先去了解一下PT,BT 前言：五一PT站PThome开放注册了，一直好奇PT的我就注册了一个账号... 喜滋滋的注册了以后，以为自此资源就不用烦了，却发现... 竟然有新手考核这种东西！ 新手考核要求做种率达到5.0,上传和下载分别达到50G,魔力值达到3000. 其中上……

Tutorial on installing texture packs of Chrome Dino Step 1 Open Chrome Dino anywhere. from chrome://dino maybe Step 2 Press F12, turn to Console and input: Runner.imageSprite.src="<Your image path>" with quotes Note that your image path shou……

博客的基本设置完成后，就折腾起了注册，然后发现默认注册需要邮件验证... 懒得改源码了上网一查网易和qq邮箱都有免费SMTP服务 (SMTP) 想了想用网易的吧 qq邮箱没逼格 网易用手机验证开通了授权码就可以使用smtp服务了 然后在添加了个邮件服务。 本以为万事大吉了，……