思考题 from Maths homework again... 数学小题两道 Problem A You are given a bipartite graph G, find a proper coloring of the complement of G that uses minimum colors. |G|\leq 100 Problem B Given integer N. Find any n\geq N such that n+\varphi(n) is ……

呃呃，笔者最大流水平真是哈哈了，请见本题： 104871C 一眼网络流，怎么构图？ Hint:有费用的网络但是不是最小费用流？那就考虑一下最小割吧！ 一个蛋糕可以考虑成：选择蛋糕->选择工具。一个蛋糕被创造需要：选择蛋糕、选择所有工具。「所有」二字让我们考虑最小割！……

题目：给出一个圆和两点，求这两点间最短路线的距离，要求路线经过圆内部或边上的任意一点。 链接：104871G 如果两个点有一个在圆内（上）就好了…… 如果两个都在圆外，设经过的圆上一点有仰角\alpha，那么注意到答案关于\alpha一定只有一个极小值。就可以三分了！ 难……

欢迎来到YZHT系列，这个系列我将分享我遇到的OI好题。 第一题 笔者最近正在学习数学图论，但是在OI中正巧碰到了这样一道从来没见过的最大流题。虽然难度不大，但是思路比较新： Problem B of 2023-2024 ICPC Southwestern European Regional Contest (SWERC 2023) 顺……

概括 原文为英文，本文是回忆版。考完收试卷、草稿纸。可以携带一张A4手写笔记。g=9.8m/s^2 ▲The Dog of Yachong Guo P1 有一个半径R质量均匀的实心球在表面产生了a_g的引力加速度。求：球内和球外加速度分别为\frac{a_g}{3}的位置。 P2 一个小球拴在L=1.25m的轻质绳……

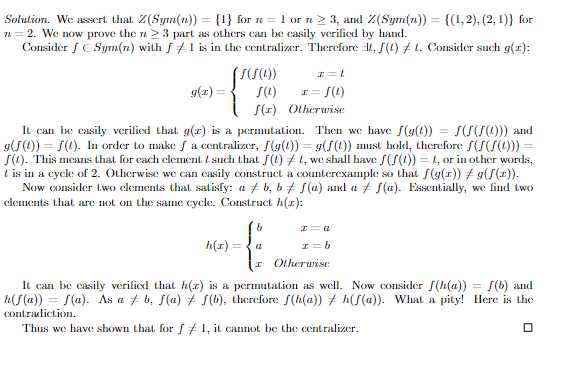
Solved! Difficulty: Div2C :O 竟然有60期了！！ 本期题目来源于Group Theory作业，，，难度不高 Congratulations on the 60th entry!! This problem is from my Group Theory homework (not very hard). Z(Sym(n))=ONE In the world of MATHS, a permutation i……

题目背景 证明连续性时缩放水平哈哈了(sweatgrinning) 题面 f is a nonconstant holomorphic function on a region \Omega_1 and g is defined on f(\Omega_1). Prove that if h=g\circ f is holomorphic, then so is g. 先想再看提示哦 提……

思考题 aimed for inexperienced participants? Silenced! Ookami and her teammates are taking an English class. The team has n people(animals?) in total. Initially, each person can raise his/her hand and speak freely. However, once a student has raise……

Solved! Difficulty: Div2 C 思考题 in mid autumn LianLi Branches Wulpit has drawn n nodes(indexed from 1 to n) and n-m edges on paper for homework assignments. They form m rooted trees (aka a forest). However, when she went to the toilet, Fely……

题目背景 完整题解来自ox帅气lecturer, 我只能证明F<\mathbb C的情况 ;( ;( ;() 题面 V是域F上的一个线性空间, \dim V=n, 且有T\in L(V). 现取\lambda_1,...,\lambda_n\in F两两不等, 且任意\lambda_i都不是T的特征值. 试证明存在\alpha_1,...,\alpha_n\in F使得 \……