Orange Boy Can You Solve It Out? Ep. 60
Solved!
Difficulty: Div2C
:O 竟然有60期了!! 本期题目来源于Group Theory作业,,,难度不高
Congratulations on the 60th entry!! This problem is from my Group Theory homework (not very hard).
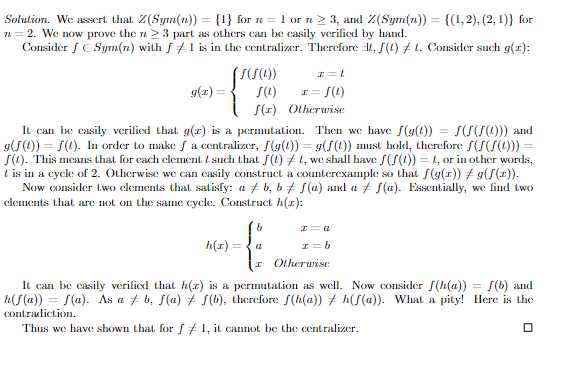
Z(Sym(n))=ONE
In the world of MATHS, a permutation is a bijection f: {1,2,3,...,n}\to {1,2,3,...,n}.
For example for n=4:
- Permutation: f(1)=2,f(2)=4,f(3)=3,f(4)=1 is a permutation.
-
but f(1)=5,f(2)=4,f(3)=3,f(4)=1 and f(1)=2,f(2)=4,f(3)=2,f(4)=1 are NOT.
Now, Ninetail has a permutation f(x) of order n and you want to get naughty. Find any permutation g(x) such that f(g(x))\neq g(f(x)). If there are multiple answers, print any. If there are none, print NO.
Examples
Input
f(1)=2,f(2)=3,f(3)=1,f(4)=4,f(5)=5
Output
g(1)=3,g(2)=2,g(3)=1,g(4)=4,g(5)=5
Explain
f(g(x))=1,3,2,4,5
g(f(x))=2,1,3,4,5
Input
f(1)=2,f(2)=1
Output
NO
Constraints
n\leq 10^6
Solutions
Solution By XGN
摸了,,,希望做法不假
版权声明:
作者:XGN
链接:https://blog.hellholestudios.top/archives/1385
来源:Hell Hole Studios Blog
文章版权归作者所有,未经允许请勿转载。

共有 0 条评论